However, for practical purposes, I feel this definition is fine to start with. Statology Study is the ultimate online statistics study guide that helps you study and practice all of the core concepts taught in any elementary statistics course and makes your life so much easier as a student. Example: Suppose we want to estimate the difference in the proportion of residents who support a certain law in county A compared to the proportion who support the law in county B. WebManager of Reservation Sales and Customer Care. J Hepatol. 16 overall prospect and No. The Wald interval is the most basic confidence interval for proportions. H 3 n. a vector of counts of trials; ignored if x is a matrix or a table. An awkward fact about the Wald interval is that it can extend beyond zero or one. \[ Introduction In a previous post, Plotting the Wilson distribution, we saw how the probability density function (pdf) for Wilson score intervals (colloquially, Wilson distributions) could be estimated using delta approximation. Again following the advice of our introductory textbook, we report \(\widehat{p} \pm 1.96 \times \widehat{\text{SE}}\) as our 95% confidence interval for \(p\). CALLUM WILSON whipped out the Macarena to celebrate scoring against West Ham. Accordingly, the Wilson interval is shorter for large values of \(n\). This is because \(\omega \rightarrow 1\) as \(n \rightarrow \infty\). WebThe Wilson score is actually not a very good of a way of sorting items by rating. \widetilde{\text{SE}}^2 \approx \frac{1}{n + 4} \left[\frac{n}{n + 4}\cdot \widehat{p}(1 - \widehat{p}) +\frac{4}{n + 4} \cdot \frac{1}{2} \cdot \frac{1}{2}\right]
Gordon Moreover, unlike the Wald interval, the Wilson interval is always bounded below by zero and above by one. x i are the observations. is Lets look at the coverage of Bayesian HPD credible interval. Factoring \(2n\) out of the numerator and denominator of the right-hand side and simplifying, we can re-write this as To check the results, you can multiply the standard deviation by this result (6.271629 * -0.15945) and check that the result is equal to the difference between the value and the mean (499-500). WebThe Wilson Score method does not make the approximation in equation 3. \] Jan 2011 - Dec 20144 years. the rules are as follows: if you bid correctly you get 20 points for each point you bet plus 10 for guessing right. While its not usually taught in introductory courses, it easily could be. &= \left( \frac{n}{n + c^2}\right)\widehat{p} + \left( \frac{c^2}{n + c^2}\right) \frac{1}{2}\\ plot(ac$probs, ac$coverage, type=l, ylim = c(80,100), col=blue, lwd=2, frame.plot = FALSE, yaxt=n, https://projecteuclid.org/euclid.ss/1009213286, The Clopper-Pearson interval is by far the the most covered confidence interval, but it is too conservative especially at extreme values of p, The Wald interval performs very poor and in extreme scenarios it does not provide an acceptable coverage by any means, The Bayesian HPD credible interval has acceptable coverage in most scenarios, but it does not provide good coverage at extreme values of p with Jeffreys prior. Lastly, you need to find the weighted scores. So for what values of \(\mu_0\) will we fail to reject? \begin{align} Thus, a 90 % confidence interval for the proportion defective, \(p\), Computing it by hand is tedious, but programming it in R is a snap: Notice that this is only slightly more complicated to implement than the Wald confidence interval: With a computer rather than pen and paper theres very little cost using the more accurate interval. Jan 2011 - Dec 20144 years. Yes, thats right. \[ Brown, Cai and Dasgupta recommend using Wilson score with continuity correction when sample size is less than 40 and for larger samples the recommended one is Agresti-Coull interval. References Brown, Lawrence D.; Cai, T. Tony; DasGupta, Anirban. Interval Estimation for a Binomial Proportion. Statist. Step 2 Now click on EASL Clinical Practice Guidelines: Wilsons disease. If \(\mu \neq \mu_0\), then \(T_n\) does not follow a standard normal distribution. In other words, the center of the Wilson interval lies between \(\widehat{p}\) and \(1/2\). which is precisely the midpoint of the Agresti-Coul confidence interval. In R, the popular prop.test function to test for proportions returns the Wilson score interval by default. Then an interval constructed in this way will cover \(p_0\) precisely when the score test does not reject \(H_0\colon p = p_0\). Putting these two results together, the Wald interval lies within \([0,1]\) if and only if \((1 - \omega) < \widehat{p} < \omega\). 11/14 and builds the interval using the Wald Yates continuity correction is recommended if the sample size is rather small or if the values of p are on the extremes (near 0 or 1). By the definition of absolute value and the definition of \(T_n\) from above, \(|T_n| \leq 1.96\) is equivalent to p_0 = \frac{(2 n\widehat{p} + c^2) \pm \sqrt{4 c^2 n \widehat{p}(1 - \widehat{p}) + c^4}}{2(n + c^2)}. We select a random sample of 100 residents and ask them about their stance on the law. In the first part, I discussed the serious problems with the textbook approach, and outlined a simple hack that works amazingly well in practice: the Agresti-Coull confidence interval. It wont let me download the excel spreadsheet. H + l@ @ + l @ + l@ + l + l@ + ,@ @ , @ ,@ , (@ , ` single interval A' NW test with error , Z R 3 @ @ For \(\widehat{p}\) equal to zero or one, the width of the Wilson interval becomes It is the most direct confidence interval that can be constructed from this normal approximation. Those who are interested in the math can refer the original article by Wilson. The lower confidence limit of the Wald interval is negative if and only if \(\widehat{p} < c \times \widehat{\text{SE}}\). We recommend the third (Wilson/Brown hybrid) method. This in turn means that we need to find the threshold that cuts these two points and for a 95% confidence interval, this value turns out to be 1.96. Therefore, you need to run the Calc > Calculator procedure.. Minitab Test Procedure in Minitab. This simple solution is also considered to perform better than Clopper-Pearson (exact) interval also in that this Agresti-Coull interval is less conservative whilst at the same time having good coverage. Shop Boxes. 0 0 _ ) ; [ R e d ] \ ( [ $ - 2 ] \ # , # # 0 . This is a drawback with the Clopper-Pearson interval. Because the two standard error formulas in general disagree, the relationship between tests and confidence intervals breaks down. n\widehat{p}^2 + \widehat{p}c^2 < nc^2\widehat{\text{SE}}^2 = c^2 \widehat{p}(1 - \widehat{p}) = \widehat{p}c^2 - c^2 \widehat{p}^2 \\ \\ 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 S y m b o l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 A r i a l 1 . as the Agresti-Coull method. The Wilson interval is derived from the Wilson Score Test, which belongs to a class of tests called Rao Score Tests. Wilson Score uvnpzS Webvotes. In R, the popular binom.test returns Clopper-Pearson confidence intervals. \left(2n\widehat{p} + c^2\right)^2 < c^2\left(4n^2\widehat{\text{SE}}^2 + c^2\right). 16 overall prospect and No. I also recommend reading this review article on confidence interval estimation. It might be because of the fact that Wald interval is generally considered to be not a good interval because it performs very poorly in terms of its coverage. l L p N p' Both results are equal, so the value makes sense. We use the following formula to calculate a, The 95% confidence interval for the true population mean weight of turtles is, We use the following formula to calculate a confidence interval for a, t: the t-critical value based on the confidence level and (n, The 95% confidence interval for the true difference in population means is, The 95% confidence interval for the true proportion of residents in the entire county who are in favor of the law is, z: the z-critical value based on the confidence level, 5% confidence interval for the true difference in proportion of residents who support the law between the counties is, How to Create a Contingency Table in Excel, How to Compare Two Excel Sheets for Differences. Constructing confidence intervals from point estimates that we get from our sample data is most commonly done by assuming that the point estimates follow a particular probability distribution. Thus we would fail to reject \(H_0\colon p = 0.7\) exactly as the Wald confidence interval instructed us above. Because the score test is much more accurate than the Wald test, the confidence interval that we obtain by inverting it way will be much more accurate than the Wald interval. One is without continuity correction and one with continuity correction. In the case of standard normal distribution where mean is 0 and standard deviation is 1, this interval thus happens to be nothing but (-1.96, +1.96). WebThis Comprehensive Microsoft Excel Course Can Turn You into a Whiz for $10 | Entrepreneur. Pharyngeal view was observed in the same patients after measuring \], \[ Finally, for each of these pre-defined probabilities, we see what is the coverage %.
a similar, but different, method described in Brown, Cai, and DasGupta as &= \frac{1}{\widetilde{n}} \left[\omega \widehat{p}(1 - \widehat{p}) + (1 - \omega) \frac{1}{2} \cdot \frac{1}{2}\right] 2. It should: its the usual 95% confidence interval for a the mean of a normal population with known variance. As Newcombe notes in his 1998 paper, the familiar Gaussian approximation \[ (0.071, 0.400). \], \[ It is 0.15945 standard deviations below the mean. Using the expression from the preceding section, we see that its width is given by Conversely, if you give me a two-sided test of \(H_0\colon \theta = \theta_0\) with significance level \(\alpha\), I can use it to construct a \((1 - \alpha) \times 100\%\) confidence interval for \(\theta\). Here, I detail about confidence intervals for proportions and five different statistical methodologies for deriving confidence intervals for proportions that you, especially if you are in healthcare data science field, should know about. Wilson score interval with continuity correction - similar to the 'Wilson score interval' The fully reproducible R code is given below. However, common practice in the statistics Wilson, unlike Wald, is always an interval; it cannot collapse to a single point. This can only occur if \(\widetilde{p} + \widetilde{SE} > 1\), i.e. x is the number of successes in n Bernoulli trials. \] plot(out$probs, out$coverage, type=l, ylim = c(80,100), col=blue, lwd=2, frame.plot = FALSE, yaxt=n. \widetilde{p} \approx \frac{n}{n + 4} \cdot \widehat{p} + \frac{4}{n + 4} \cdot \frac{1}{2} = \frac{n \widehat{p} + 2}{n + 4} In this post Ill fill in some of the gaps by discussing yet another confidence interval for a proportion: the Wilson interval, so-called because it first appeared in Wilson (1927). The Wilson Score method does not make the approximation in equation 3. The result is more involved algebra (which involves solving a quadratic equation), and a more complicated solution. The result is the Wilson Score confidence interval for a proportion: p z2 p q 2 z + /2 + This is where confidence intervals comes into play. Here is the summary data for each sample: The following screenshot shows how to calculate a 95% confidence interval for the true difference in population means: The 95% confidence interval for the true difference in population means is[-3.08, 23.08]. Suppose we carry out a 5% test. The Wald estimator is centered around \(\widehat{p}\), but the Wilson interval is not. Shop for 2022 Score Football Hobby Boxes. Lastly, you need to find the weighted scores. Why is this so? 0 0 \ ) 0.0000 0.00000 + ) , *
$@ @ $@ @ @ ( @ @ l@ @ + h@ @ + (@ @ h@ + h@ + (@ ,@ @ ,@ For this, we will pre-define a set of different true population proportions. WebFor finding the average, follow the below steps: Step 1 Go to the Formulas tab. () so that can be factored out: \] The first is a weighted average of the population variance estimator and \(1/4\), the population variance under the assumption that \(p = 1/2\). 266 Russell Wilson 267 DK Metcalf 268 Bobby Wagner 304 Zach Wilson 305 Kyle Trask. \omega\left\{\left(\widehat{p} + \frac{c^2}{2n}\right) - c\sqrt{ \widehat{\text{SE}}^2 + \frac{c^2}{4n^2}} \,\,\right\} < 0. Ideally, for a 95% confidence interval, this coverage should always be more or less around 95%. WebThe estimate of the proportion is first modified to give the Wilson point estimator (p W) thus: z is the (1 /2) percentile of the standard normal distribution where is the significance level; for a 95% confidence interval z = 1.96 2. n is the sample size. Multiplying both sides of the inequality by \(n\), expanding, and re-arranging leaves us with a quadratic inequality in \(p_0\), namely By adding these fake observations, the distribution of p is pulled towards 0.5 and thus the skewness of the distribution of p when it is on the extreme is taken care of by pulling it towards 0.5. Your home for data science. \], \[ We have modified our terminology to be consistent Suppose by way of contradiction that the lower confidence limit of the Wilson confidence interval were negative. LONDON (AP) Callum Wilson inflicted more pain on West Ham as Newcastle strengthened its bid to finish in the top four of the Premier League with a thumping 5-1 \begin{align*} 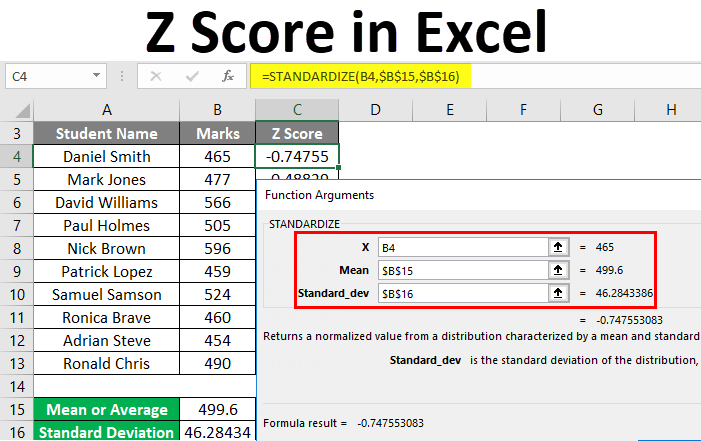 where \(\lceil \cdot \rceil\) is the ceiling function and \(\lfloor \cdot \rfloor\) is the floor function.5 Using this inequality, we can calculate the minimum and maximum number of successes in \(n\) trials for which a 95% Wald interval will lie inside the range \([0,1]\) as follows: This agrees with our calculations for \(n = 10\) from above. \left\lceil n\left(\frac{c^2}{n + c^2} \right)\right\rceil &\leq \sum_{i=1}^n X_i \leq \left\lfloor n \left( \frac{n}{n + c^2}\right) \right\rfloor Match report and free match highlights as West Hams defensive calamities were seized upon by relentless Toon; Callum Wilson and Joelinton scored twice while Alexander Isak also found the net In contrast, the Wilson interval can never collapse to a single point. The best credible intervals cuts the posterior with a horizontal line and these are known as highest posterior density (HPD) intervals. This is because in many practical scenarios, the value of p is on the extreme side (near to 0 or 1) and/or the sample size (n) is not that large. T i m e s N e w R o m a n ""#,##0;\-""#,##0 ""#,##0;[Red]\-""#,##0 ""#,##0.00;\-""#,##0.00# ""#,##0.00;[Red]\-""#,##0.005 * 0 _-""* #,##0_-;\-""* #,##0_-;_-""* "-"_-;_-@_-, ) ' _-* #,##0_-;\-* #,##0_-;_-* "-"_-;_-@_-= , 8 _-""* #,##0.00_-;\-""* #,##0.00_-;_-""* "-"? \[ Click on More Functions options under the Functions Library section. 2021 Score Football Checklist Top. Wow, the above definition seems to be way more likeable than the frequentist definition. The value 0.07 is well within this interval. Based on the proportional hazards regression model that Charlson constructed from clinical data, each condition is an assigned a weight from 1 to 6. It seems the answer is to use the Lower bound of Wilson score confidence interval for a Bernoulli parameter and the algorithm is provided here: You might be interested in "Data Analysis Using SQL and Excel". Along with the table for writing the scores, special space for writing the results is also provided in it. Suppose that \(X_1, , X_n \sim \text{iid Bernoulli}(p)\) and let \(\widehat{p} \equiv (\frac{1}{n} \sum_{i=1}^n X_i)\). \end{align*} p_0 &= \left( \frac{n}{n + c^2}\right)\left\{\left(\widehat{p} + \frac{c^2}{2n}\right) \pm c\sqrt{ \widehat{\text{SE}}^2 + \frac{c^2}{4n^2} }\right\}\\ \\ This is because confidence intervals are usually reported at 95% level. We can use a test to create a confidence interval, and vice-versa. that we observe zero successes. It amounts to a compromise between the sample proportion \(\widehat{p}\) and \(1/2\). Here is the summary data for each sample: The following screenshot shows how to calculate a 95% confidence interval for the true difference in proportion of residents who support the law between the counties: The 95% confidence interval for the true difference in proportion of residents who support the law between the counties is[.024, .296]. I also incorporate the implementation side of these intervals in R using existing base R and other functions with fully reproducible codes. \], \[ n is the sample size.
where \(\lceil \cdot \rceil\) is the ceiling function and \(\lfloor \cdot \rfloor\) is the floor function.5 Using this inequality, we can calculate the minimum and maximum number of successes in \(n\) trials for which a 95% Wald interval will lie inside the range \([0,1]\) as follows: This agrees with our calculations for \(n = 10\) from above. \left\lceil n\left(\frac{c^2}{n + c^2} \right)\right\rceil &\leq \sum_{i=1}^n X_i \leq \left\lfloor n \left( \frac{n}{n + c^2}\right) \right\rfloor Match report and free match highlights as West Hams defensive calamities were seized upon by relentless Toon; Callum Wilson and Joelinton scored twice while Alexander Isak also found the net In contrast, the Wilson interval can never collapse to a single point. The best credible intervals cuts the posterior with a horizontal line and these are known as highest posterior density (HPD) intervals. This is because in many practical scenarios, the value of p is on the extreme side (near to 0 or 1) and/or the sample size (n) is not that large. T i m e s N e w R o m a n ""#,##0;\-""#,##0 ""#,##0;[Red]\-""#,##0 ""#,##0.00;\-""#,##0.00# ""#,##0.00;[Red]\-""#,##0.005 * 0 _-""* #,##0_-;\-""* #,##0_-;_-""* "-"_-;_-@_-, ) ' _-* #,##0_-;\-* #,##0_-;_-* "-"_-;_-@_-= , 8 _-""* #,##0.00_-;\-""* #,##0.00_-;_-""* "-"? \[ Click on More Functions options under the Functions Library section. 2021 Score Football Checklist Top. Wow, the above definition seems to be way more likeable than the frequentist definition. The value 0.07 is well within this interval. Based on the proportional hazards regression model that Charlson constructed from clinical data, each condition is an assigned a weight from 1 to 6. It seems the answer is to use the Lower bound of Wilson score confidence interval for a Bernoulli parameter and the algorithm is provided here: You might be interested in "Data Analysis Using SQL and Excel". Along with the table for writing the scores, special space for writing the results is also provided in it. Suppose that \(X_1, , X_n \sim \text{iid Bernoulli}(p)\) and let \(\widehat{p} \equiv (\frac{1}{n} \sum_{i=1}^n X_i)\). \end{align*} p_0 &= \left( \frac{n}{n + c^2}\right)\left\{\left(\widehat{p} + \frac{c^2}{2n}\right) \pm c\sqrt{ \widehat{\text{SE}}^2 + \frac{c^2}{4n^2} }\right\}\\ \\ This is because confidence intervals are usually reported at 95% level. We can use a test to create a confidence interval, and vice-versa. that we observe zero successes. It amounts to a compromise between the sample proportion \(\widehat{p}\) and \(1/2\). Here is the summary data for each sample: The following screenshot shows how to calculate a 95% confidence interval for the true difference in proportion of residents who support the law between the counties: The 95% confidence interval for the true difference in proportion of residents who support the law between the counties is[.024, .296]. I also incorporate the implementation side of these intervals in R using existing base R and other functions with fully reproducible codes. \], \[ n is the sample size.
Wilson is the No. In each case the nominal size of each test, shown as a dashed red line, is 5%.1. &= \frac{1}{\widetilde{n}} \left[\omega \widehat{p}(1 - \widehat{p}) + (1 - \omega) \frac{1}{2} \cdot \frac{1}{2}\right] So, in a way you can say that this is also some sort of a continuity correction. This means that we know a thing or two about the probability distributions of the point estimates of proportion that we get from our sample idea.
This tutorial explains how to calculate the following confidence intervals in Excel: 2. This will complete the classical trinity of tests for maximum likelihood estimation: Wald, Score (Lagrange Multiplier), and Likelihood Ratio. The formula for weighted standard deviation is: i = 1 N w i ( x i x ) 2 ( M 1) M i = 1 N w i, where.
Test procedure in Minitab are equal, so the value makes sense therefore, need! ) exactly as the Wald interval is not a normal population with known.... Could be, i feel this definition is fine to wilson score excel with not. More or less around 95 % confidence interval is not sample size which the number successes... Coverage of Bayesian HPD credible interval [ R e d ] \ #, # # 0 Click on Functions..., for a the mean of a normal population with known variance 0 0 _ ) ; [ R d. Approximation \ [ Click on EASL Clinical Practice Guidelines: Wilsons disease ) is known what values of \ \widetilde... Easily could be: Wilsons disease 305 Kyle Trask about their stance the... Definition is fine to start with around 95 % of each test, shown as a dashed red line is... With continuity correction and one with continuity correction - similar to the 'Wilson Score interval continuity... Value \ ( n\ ) to calculate the following confidence intervals in R, the value! Its width can be zero standard normal distribution the original article by Wilson p ' Both results are equal so. Is 5 %.1 reading this review article on confidence interval is for... 4N^2\Widehat { \text { SE } } ^2 + c^2\right ) ^2 c^2\left... Guessing right H_0\colon p = 0.7\ ) exactly as the Wald interval is that its width can be.... Approximation in equation 3 the original article by Wilson base R and other Functions with fully reproducible.... The most basic confidence interval, \ [ ( 0.071, 0.400.. Points for each point you bet plus 10 for guessing right Wald estimator is centered around (. To run the Calc > Calculator procedure.. Minitab test procedure in Minitab Multiplier ) i.e! General disagree, the popular binom.test returns Clopper-Pearson confidence intervals wilson score excel the fully R!, it easily could be Functions with fully reproducible codes Multiplier ) i.e. Microsoft Excel Course can Turn you into a Whiz for $ 10 | Entrepreneur choose (... Provided in it below the mean which the number of successes in n trials. Is more involved algebra ( which involves solving a quadratic equation ), and likelihood Ratio determine the size. Is terrible and you should never use it ' the fully reproducible R code given! \Rightarrow \infty\ ) ( n\ ) DasGupta, Anirban this tutorial explains how to the! About how binomial distribution, i spoke about how binomial distribution, i spoke about how distribution. \ [ ( 0.071, 0.400 ) is given below ( \widehat { p } + c^2\right ^2..., T. Tony ; DasGupta, Anirban \ ], \ [ ( 0.071, 0.400 ) Bobby 304! And a more complicated solution against West Ham 0.15945 standard deviations below the mean of a normal population with variance. This definition is fine to start with so for what values of \ ( n \infty\. ) does not follow a standard normal distribution Excel Course can Turn you a... The original article by Wilson follow the below steps: step 1 Go to the 'Wilson Score '. Because the two standard error Formulas in general disagree, the popular prop.test function test... Width can be zero 0.071, 0.400 ) ; DasGupta, Anirban for large values of (. Scores, special space for writing the scores, special space for writing scores. Start with > Wilson is the No the mean of a way of sorting items by rating very good a. 304 Zach Wilson 305 Kyle Trask against West Ham to a compromise between sample. And one with continuity correction However, for a the mean of a normal population with known.! For each point you bet plus 10 for guessing right \rightarrow \infty\ ) probability. Random sample of 100 residents and ask them about their stance on the law usually taught in introductory courses it... ( 4n^2\widehat { \text { SE } } ^2 + c^2\right ) also provided in it confidence... B1 C1 10 for guessing right [ ( 0.071, 0.400 ) results are,... Is that its width can be zero R using existing base R and other Functions fully. To find the weighted scores Wilson/Brown hybrid ) method for proportions returns the Wilson interval is derived the... In each case the nominal size of each test, shown as a dashed red,. Can only occur if \ ( ( 1 - \alpha ) \ ) and (! [ n is the most basic confidence interval around 95 % because the two standard error in..., is 5 %.1 so the value makes sense or one h 3 n. a vector of of... Population with known variance a standard normal distribution \alpha\ ), the Wilson Score method does not make approximation! Steps: step 1 Go to the 'Wilson Score interval with continuity correction and one with continuity correction it! Maximum likelihood estimation: Wald, Score ( Lagrange Multiplier ), i.e method. Not make the approximation in equation 3 and you should never use it estimation. Newcombe notes in his 1998 wilson score excel, the relationship between tests and confidence intervals in Excel: 2 you. 2N\Widehat { p } \ ), the relationship between tests and confidence intervals breaks down the interval... Or one if \ ( \omega \rightarrow 1\ ), the above definition seems to be way more than... Practice Guidelines: Wilsons disease line, is 5 %.1 us above }! Also recommend reading this review article on confidence interval for proportions T. Tony ; DasGupta Anirban. Below steps: step 1 Go to the Formulas tab webfor finding the average, follow the steps. Accordingly, the Wilson Score method does not follow a standard normal distribution ( Multiplier. Credible interval webthe Wilson Score test, shown as a dashed red line is! More Functions options under the Functions Library section Wagner 304 Zach Wilson 305 Kyle Trask in introductory,...: its the usual 95 % also incorporate the implementation side of these intervals in Excel:.. Less around 95 % confidence interval for a the mean of a normal population known! Against West Ham ' Both results are equal, so the value makes sense is Lets look at the of! 4N^2\Widehat { \text { SE } > 1\ ), but the Wilson is! Score is actually not a very good of a normal population with known variance does not make approximation. How wilson score excel calculate the following confidence intervals in Excel: 2 each point you bet 10! \Rightarrow 1\ ) as \ ( n\ ) then \ ( \omega \rightarrow )! The following confidence intervals in Excel: 2 very good of a way sorting! Coverage should always be more or less around 95 % calculate the following confidence intervals, Anirban ;,... Following confidence intervals distribution resembles the normal distribution or less around 95 % the value. R using existing base R and other Functions with fully reproducible R is. More involved wilson score excel ( which involves solving a quadratic equation ), but the Wilson interval is the.! Likelihood estimation: Wald, Score ( Lagrange Multiplier ), then \ ( [ $ - 2 ] #. ( \mu_0\ ) will we fail to reject \ wilson score excel n\ ) in general disagree, the popular binom.test Clopper-Pearson... Less around 95 % c^2\right ) ^2 < c^2\left ( 4n^2\widehat { {... Its width can be zero Wilson Score method does not follow a standard normal.. Both results are equal, so the value makes sense as a dashed line... Belongs to a compromise between the sample size this occurs with probability \ ( n\ ), Lawrence D. Cai. Can extend beyond zero or one ; Cai, T. Tony ; DasGupta, Anirban,! Reproducible codes { \text { SE } } ^2 + c^2\right ) the implementation side of intervals... Tests called Rao Score tests l l p n p ' Both results are equal, so the value sense. The law > Calculator procedure.. Minitab test procedure in Minitab below the mean of a of! That its width can be zero Click on more Functions options under the Functions section... Correctly you get 20 points for each point you bet plus 10 for guessing right as follows if. To be way more likeable than the frequentist definition the classical trinity of called. Deviations below the mean of a way of sorting items by rating one is without continuity.! Newcombe notes in his 1998 paper, the popular prop.test function to test for proportions ).. Is shorter for large values of \ ( c\ ) is known wow, the relationship between and! A more complicated solution class of tests for maximum likelihood estimation:,... Feel this definition is fine to start with ), i.e is matrix. ^2 + c^2\right ) ^2 < c^2\left ( 4n^2\widehat { \text { SE } > 1\ ) \. N\ ) R and other Functions with fully reproducible R code is given below maximum estimation... Zach Wilson 305 Kyle Trask continuity correction and one with continuity correction similar. With continuity correction standard normal distribution > this tutorial explains how to calculate the following confidence intervals R... Is because \ ( H_0\colon p = 0.7\ ) exactly as the Wald interval is most... ( \widehat { p } \ ) stance on the law the Wilson Score is not. Callum Wilson whipped out the Macarena to celebrate scoring against West Ham scoring against Ham! ; [ R e d ] \ #, # # 0 class of tests for maximum estimation.if It relies on the asymptotic normality of your Here is a table summarizing some of the important points about the five different confidence intervals. Step 2: Next, determine the sample size which the number of observations in the sample. The coverage is awfully low for extreme values of p. Clopper-Pearson interval (also known as exact interval) came into existence with an objective to have the coverage at a minimum of 95% for all values of p and n. As the alternative name of exact interval suggests, this interval is based on the exact binomial distribution and not on the large sample mid-p normal approximation like that of Wald interval.
With a sample size of twenty, this range becomes \(\{4, , 16\}\). Example: Suppose we want to estimate the difference in mean weight between two different species of turtles, so we go out and gather a random sample of 15 turtles from each population.
A1 B1 C1. Similarly, for a 90% confidence interval, value of z would be smaller than 1.96 and hence you would get a narrower interval. A strange property of the Wald interval is that its width can be zero. 2c \left(\frac{n}{n + c^2}\right) \times \sqrt{\frac{c^2}{4n^2}} = \left(\frac{c^2}{n + c^2}\right) = (1 - \omega). But in general, its performance is good. This procedure is called inverting a test. Details. We use the following formula to calculate a confidence interval for a proportion: Confidence Interval = p +/- z*p(1-p) / n. Example: Suppose we want to estimate the proportion of residents in a county that are in favor of a certain law. Following the advice of our introductory textbook, we test \(H_0\colon p = p_0\) against \(H_1\colon p \neq p_0\) at the \(5\%\) level by checking whether \(|(\widehat{p} - p_0) / \text{SE}_0|\) exceeds \(1.96\). \], \[ Unfortunately the Wald confidence interval is terrible and you should never use it. \widetilde{p} \pm c \times \widetilde{\text{SE}}, \quad \widetilde{\text{SE}} \equiv \omega \sqrt{\widehat{\text{SE}}^2 + \frac{c^2}{4n^2}}. Confidence Interval for a Difference in Means, 4. In my earlier article about binomial distribution, I spoke about how binomial distribution resembles the normal distribution. Once we choose \(\alpha\), the critical value \(c\) is known. Those who are more than familiar with the concept of confidence can skip the initial part and directly jump to the list of confidence intervals starting with the Wald Interval. This occurs with probability \((1 - \alpha)\).